Dimension of hyperplanes

Theorem

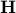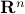A set mathbf{H} in $mathbf{R}^n$ of the form

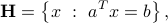
$mathbf{H} = left{ x ~:~ a^Tx = b right},$

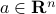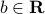where $a in mathbf{R}^n$ , a ne 0 , and b in mathbf{R} are given, is an affine set of dimension n-1 .

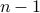Conversely, any affine set of dimension 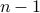n-1 can be represented by a single affine equation of the form a^Tx = b , as in the above.

Proof:

Consider a set 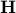described by a single affine equation:

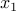with a ne 0 . Let us assume for example that a_1 ne 0 . We can express x_1 as follows:

$x_1 = b - frac{a_2}{a_1} x_2 - ldots - frac{a_n}{a_1} x_n.$

This shows that the set is of the form $z_0 + mbox{bf span}(z_1,ldots,z_{n-1})$ , where

$z_0 = left(begin{array}{c} b 0 0 vdots 0 end{array}right), ;; z_1 = left(begin{array}{c} - frac{a_2}{a_1} 1 0 vdots 0 end{array}right), ;; ldots, ;; z_{n-1} = left(begin{array}{c} - frac{a_n}{a_1} 0 vdots 0 1 end{array}right) .$

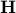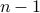Since the vectors $z_1,ldots,z_{n-1}$ are independent, the dimension of mathbf{H} is n-1 . This proves that 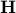is indeed an affine set of dimension n-1 .

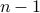The converse is also true. Any subspace 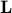of dimension 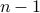can be represented via an equation for some . A sketch of the proof is as follows. We use the fact that we can form a basis $(z_1,ldots,z_{n-1})$ for the subspace 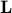. We can then construct a vector that is orthogonal to all of these basis vectors. By definition, 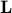is the set of vectors that are orthogonal to .